An approach for obtaining Monte Carlo computed variable proton RBE from a treatment planning system
PO-1725
Abstract
An approach for obtaining Monte Carlo computed variable proton RBE from a treatment planning system
Authors: johannes tjelta1,2, Lars Fredrik Fjæra3, Kristian Smeland Ytre-Hauge2, Camilla Boer1, Camilla Hanquist Stokkevåg1,3
1Haukeland University Hospital, Department of Oncology and Medical Physics, Bergen, Norway; 2University of Bergen , Department of Physics and Technology, Bergen, Norway; 3University of Bergen, Department of Physics and Technology, Bergen, Norway
Show Affiliations
Hide Affiliations
Purpose or Objective
Currently a constant relative biological effectiveness (RBE)
is employed during clinical proton therapy, although it is well known that the
RBE is variable depending on linear energy transfer
(LET), dose level and tissue. The lack of versatile
tools for calculation of the RBE variability is potentially degrading plan
quality and preventing fulfillment of high accuracy protons. LET
can be obtained using a Monte Carlo (MC), although calibration should
be performed to achieve correct values representative for the specific
treatment planning system (TPS). This work provides a streamlined method for
calibrating range, stopping power, density corrections, relative stopping power
and dose for the MC to treatment planning of intensity-modulated proton therapy (IMPT).
Material and Methods
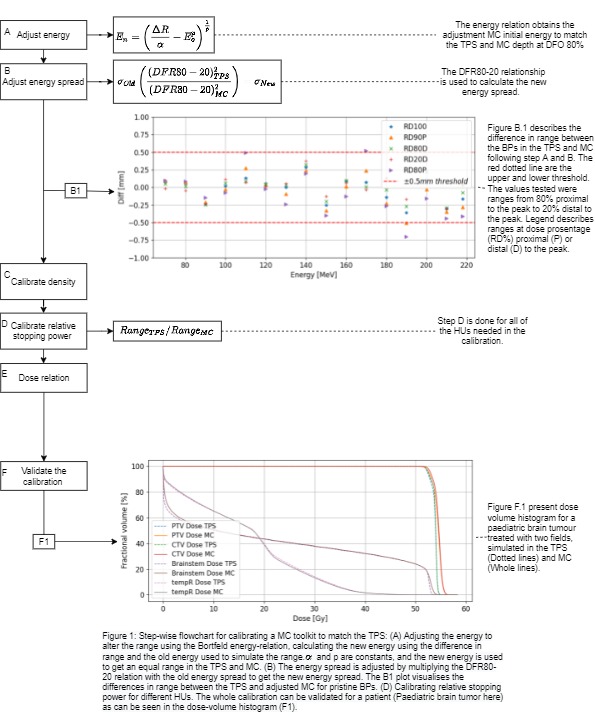
An overview of the stepwise calibration procedure is
presented in Fig 1. Pristine Bragg peaks (BPs) were created in 10 MeV intervals
in the Eclipse TPS (ProBeam360 data) as well using the FLUKA MC. A
phantom with a relative proton stopping power (RPSP)=1 was used for the TPS and
set to water for the MC. Subsequently, the MC parameters,
initial energy and energy spread were adjusted to fit the TPS: The
Bortfeld energy-range relation was rearranged for adjustment to the initial
energy of the beam and applied to fine-tune the range of the MC BPs at 80% of
the distal dose fall-off (DFO) (Fig 1.A). To modify energy spread, the distal
dose fall-off range 80%-20% (DFR80-20) of the pristine BP was calculated for
the MC and TPS. The correction factor was decided by dividing the DFR80-20 for
the TPS by the MC value (Fig 1.B1). Density corrections for the
different materials were calculated based on CT-calibration curves from the TPS
(Fig 1.C). Relative stopping power for Hounsfield units (HUs) in the interval
-925 to 3069 was calculated by dividing the range at 80% of DFO for the TPS by
the MC (Fig 1.D). Trapezoidal
integration was applied to find the relationship between the dose per particle for the MC and dose per monitor unit (MU) in the TPS. The calibration was validated
in a spread-out Bragg peak (SOBP) in water and in a 2-field treatment plan of a
pediatric brain tumor.
Results
Differences in both range and energy spread were within +/- 5 mm along the depth curve for different % of the Bragg peak (Figure 1.B.1). The
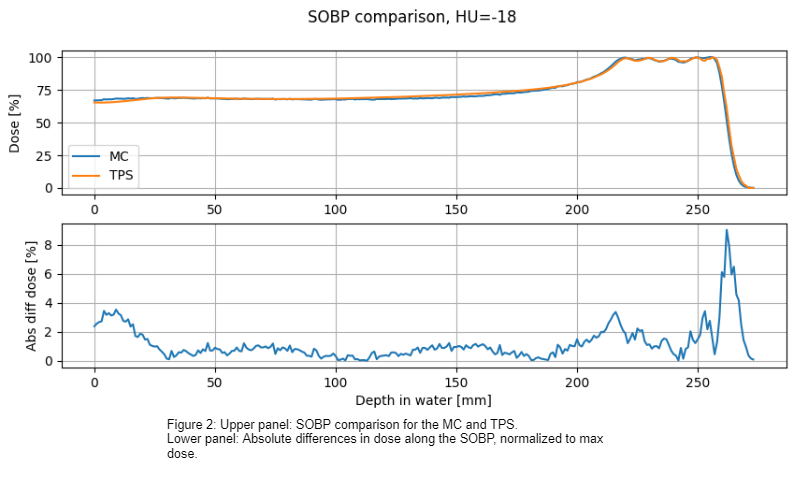
SOBP validation resulted in an absolute mean difference in dose of 1.1% for the
whole SOBP (Figure 2), and a
maximum of 9% difference in dose for the SOBP. The
patient validation resulted in good accuracy between the TPS and MC for all
dose levels, with modest differences found in the high dose regions for both target
volumes and organs at risk (Fig 1.F1).
Conclusion
An efficient
approach for calibrating MC codes to a specific TPS has been presented and
achieved good accuracy in range, energy spread, density and stopping power. The
procedure can be applied to any specific TPS
and IMPT delivery unit as well as measurements, and allows for calculating LET
values and subsequently variable RBE.